Error Function Calculator

Error Function calculator estimates the value of error function for the given a real number $x$ by using the integration formula. It is an online probability and statistics tool for data analysis programmed to estimate the relative precision of the approximation.
It is necessary to follow the next steps:

Enter a value in the box. The value must be a real number;
Press the "CALCULATE" button to make the computation;
Error function calculator will find the error function of the given real number $x$.

Input : A real number;
Output : A real number;

Error Function Formula :

The error function is a defined by the formula $$\mbox{erf}(x)=\frac{2}{\sqrt{\pi}}\int_{0}^{x}e^{-t^2}dt$$

What is Error Function?

The error function, or sometimes called the Gauss error function is a special function of sigmoid shape that occurs in probability, statistics, and partial differential equations describing diffusion. The error function is denoted by $\mbox{erf}(x)$ and it is determined by the following formula

$$\mbox{erf}(x)=\frac{1}{\sqrt{\pi}}\int_{-x}^{x}e^{-t^2}dt=\frac{2}{\sqrt{\pi}}\int_{0}^{x}e^{-t^2}dt$$

The name were proposed by J. W. L. Glaisher in 1871 on account of its connection with the theory of Probability, especially with the theory of Errors. Denoting the modulus of precision by $h$, the probability that a deviation lies between $x$ and $-x$ is

$$\mbox{erf}(hx)=\frac{2}{\sqrt{\pi}}\int_{0}^{x}e^{-h^2t^2}tdt$$

Because $e^{-t^2}$ is an even function, then

$$\mbox{erf}(-x)=- \mbox{erf}(x)$$

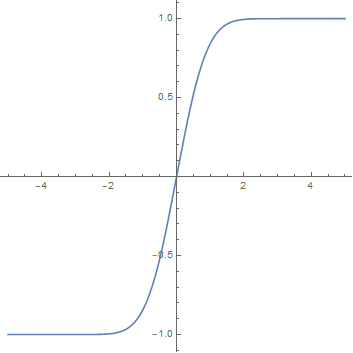
i.e. the error function is an odd function. Note that odd functions are symmetric with respect to the origin. The error function has the values

$$\mbox{erf}(0)=0,\quad \mbox{erf}(1)=+\infty$$

In the picture below is shown the error function for real values

Since

$$e^{-x^2}=1-x^2+\frac{x^4}{2!}-\frac{x^6}{3!}+\ldots$$

then

$$\begin{align}\mbox{erf}(x)&=\frac{2}{\sqrt{\pi}}\int_{0}^{x}(1-t^2+\frac{t^4}{2!}-\frac{t^6}{3!}+\ldots)dt\\ &=\frac{2}{\sqrt{\pi}}(x-\frac{x^3}{1!3}+\frac{x^5}{2!5}-\frac{x^7}{3!7}+\ldots)\end{align}$$

This series converges for all values of $x.$ So, the error function can also be defined as a Maclaurin series

$$\begin{align}\mbox{erf}(x)&=\frac{2}{\sqrt{\pi}}(x-\frac{x^3}{3}+\frac{x^5}{10}-\frac{x^7}{42}+\frac{x^9}{216}-\ldots)\end{align}$$

The error function can also be extended to the complex plane. A table of integrals of the error functions can be found on internet.

The error function calculator shows the complete step-by-step calculation for finding the error function for $x=2$ using the error function formulas. For any other values, just supply a real number and click on the "CALCULATE" button. The grade school students may use this error function calculator to generate the work, verify the results of the integral of the standard normal distribution or do their homework problems efficiently.

Error Function Practice Problems

The collection of tools employs the study of methods and procedures used for gathering, organizing, and analyzing data to understand the theory of probability and statistics. In many applications, it is necessary to calculate precision approximation, where this error function calculator can assist us to make calculation easy. In physics, the error function is used in Maxwell-Boltzmann distributions because the velocities of particles are normally distributed. The integrals of velocity distributions, give error functions and are important in various stability criteria for plasmas in thermal equilibrium.

Practice Problem 1:
Find $\mbox{erf}(5)$.

Practice Problem 2:
Evaluate integral $\frac{2}{\sqrt{\pi}}\int_5^{10}e^{-x^2}dx$.

The error function calculator, formula and practice problems would be very useful for grade school students (K-12 education) to understand the concept of the error function. This concept can be of benefit in the solutions of diffusion problems in heat, mass and momentum transfer, probability theory, the theory of errors, etc.