Weibull Distribution Calculator
Weibull Distribution - work with steps
Input Data :
Alpha (α) = 0.5
Beta (β) = 0.5
X1 = 1.5
X2 = 1
Objective :
Find what is weibull distribution for given input data?
Formula :
P(X1 < X < X2) = e(-x1/β)α - e(-x2/β)α
Solution :
= e(-1.5/2)0.5 - e(-1/2)0.5
= e(-0.75)0.5 - e(-0.5)0.5
= e(-0.866) - e(-0.7071)
= (0.4206) - (0.4931)
P(X1 < X < X2) = 0.0724
Weibull Distribution Calculator is an online probability and statistics tool for data analysis programmed to calculate precise failure analysis and risk predictions with extremely small samples using a simple and useful graphical plot. This calculator uses the input values of slope β, α and the data sets to generate the respective output values of mean, median, mode variance and standard deviation.
Definition - Weibull Distribution
Weibull Distribution offers true failure analysis and risk calculations with enormously tiny samples. Results are possible at the initial stage of a problem without the necessity to crash a few more. The Weibull distribution is a 3 factor distribution. The three factors that comprise the Weibull distribution are β, α and data sets. Weibull analysis is used widely because this distribution allows representation to be done with a negligible amount of failures. The Weibull distribution's strong point is its adaptability. Depending on the parameters' values, it can approximate an exponential, a normal or a twisted distribution.
Weibull Distribution Formula
In probability theory and statistics, the Weibull distribution is a continuous probability distribution and can be calculated from the following formula
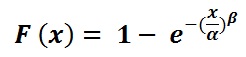
The Weibull factor B (beta) is the slope. It implies the rate of failure. The Weibull shape factor β designates whether the failure rate is increasing, constant or decreasing. When β < 1.0 designates that the product has a decreasing failure rate. This scenario is typical of infant mortality and indicates that the product is failing during its burn-in period. When β = 1.0 designates a constant failure rate. Frequently, components that have survived burn-in will subsequently exhibit a constant failure rate. The β > 1.0 designates an increasing failure rate. This is typical of products that are wearing out
The Weibull attribute life α, is a measure of the range, or spread, in the distribution of data. It therefore occurs that α equals the number of cycles at which 63.2 percent of the product has failed. In other words, for a Weibull distribution, α = 0.368, despite the value of β
The collection of tools employs the study of methods and procedures used for gathering, organizing, and analyzing data to understand theory of Probability and Statistics. The set of ideas which is intended to offer the way for making scientific implication from such resulting summarized data. In many applications it is necessary to calculate the Weibull distribution for a given sets of data. With this online calculator you can effortlessly make your statistical Weibull distribution calculation for given data sets
