Binomial Distribution Calculator

binomial distribution calculator - to estimate the probability of number of success or failure in a sequence of n independent trials or experiments. The success or failure experiment which is used in this calculator is also called as Bernoulli's experiment or distribution or trial and is the fundamental for the binomial test of statistical significance. In probability & statistics for data analysis, binomial distribution is a discrete probability function widely used method to model the number of successes and failures in n independent numbers of trials or experiments. P(x) is the probability of x successes occur in the n number of events, p is the probability of success and q is the probability of failure often denoted by q = (1 - p).
The binomial distribution arise for the following 4 conditions, when the event has
1. n identical trials or experiments
2. Two possible outcomes for each trial or experiments are success and failure.
3. Each trials or experiments are independent, e.g. each coin toss doesn't affect the others.
4. P(success) = p is the probability of success same for each trial or experiments.

Formula

The below formulas are used in this binomial distribution calculator to estimate the number of success and failures in n independent number of trials or experiments and the solved example problem illustrates how the values are being used in the formula.


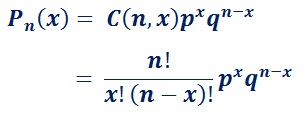
In the theory of probability & statistics, binomial distribution plays vital role to finding the probability of success in a sequence of n independent experiments. When it comes to online to verify or perform such calculations, this online binomial distribution calculator may help users to make the calculation as simple as possible. The solved example problems for binomial distribution along with step by step calculation help users to understand how the values are being used in the formula.